Close
Time turns backwards at the Spark Festival
By zcahe91, on 28 August 2015
While real time travel may be theoretically possible, it almost certainly won’t happen in our lifetimes. However, at the Spark Festival this weekend, the Chalkdust team will have a very surprising demonstration where we can turn time backwards.
For a sneak preview, watch this video of a Taylor-Couette cell (sound not necessary):
Surprised? I certainly was when I saw it for the first time.
For those who couldn’t watch the video: at first, blobs of coloured syrup are dropped into some clear syrup. Then the colours are mixed up by turning a tube inside the cylinder, until they look all brown. However, the tube is then turned back the other way, and the coloured liquid completely unmixes, ending up in the same blobs as they started. It looks exactly like the video has played backwards. It’s called reversible flow, and we’re very excited to have our own Taylor-Couette cell at the festival, where people of all ages can have a go at turning back time.
Now you might be thinking – what’s that got to do with maths?
But we can explain and predict this very weird behaviour, and lots more using fluid dynamics, the study of all things that flow. So as well as working out why we can turn back time in this case, we can also explain why aeroplanes stay in the sky, understand the growth of blood vessels in tumours, predict the weather, and work out what’s going on with a chocolate fountain.
The brilliance of it from a mathematical perspective is that just one number tells us a lot about how the fluid will behave. It’s called the Reynolds number, and it depends on several properties of our fluid:
For our experiment, the Reynolds number is very low – for a number of reasons. Firstly, the syrup is really sticky and thick, and so the viscosity is high. Here our length scale is the distance between the outer and inner cylinder, which is small, and the speed is the speed we mix up the liquid, which is again small.The reversible behaviour that we see only happens when the Reynolds number is small. This makes sense – water is much less viscous than syrup, so if we used water instead, the Reynolds number would be much bigger and we wouldn’t be able to unmix the fluids. We could also increase the Reynolds number by turning the inner tube faster, and as you might intuitively expect, the flow would no longer reverse. More surprisingly, increasing the density of the fluid or the distance between the walls would have the same effect.But why does a low Reynolds number mean we can turn back time?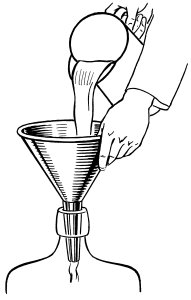
The Reynolds Number can be thought of as the ratio between the “inertial forces” and “viscous forces” in a fluid. These are both forces inside the fluid, from the fluid acting on itself. Viscous forces are friction between the fluid and itself, and the fluid and the walls, which slow down the flow. Inertial forces are slightly harder to understand, but we can think of them by considering a small section of the fluid. This small section is pushed along by the motion of the surrounding fluid, causing it to accelerate, and these are the inertial forces. For example, think about water flowing through a funnel. The water at the top moves quicker than the water coming out at the bottom because the inertial forces have pushed it along, causing it to speed up.
Now in our set up, because the Reynolds number is low, the viscous forces are much larger than the inertial forces, and so completely dominate the flow. When we turn the handle, the fluid towards the centre of the cylinder starts rotating, but the friction within the fluid slows it down as we move outwards, and as a result the coloured liquid gets stretched out into a thin ribbon of colour. Because the inertial forces are so small, there are no other effects, and when we turn it the other way, the ribbon is compressed back into the original blob. This is like pushing a ball across a table one way for two seconds, and then pushing it back the other way for two seconds. In that situation you aren’t surprised that it ends up in the same place. But most fluid flows we encounter don’t have low Reynolds number, and so inertial forces cause them to accelerate in lots more complicated ways. What’s so surprising about the Taylor-Couette cell then is the lack of inertial forces.
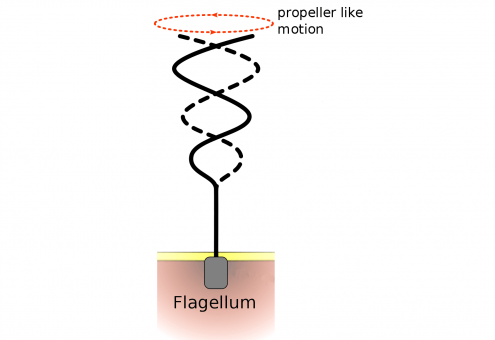
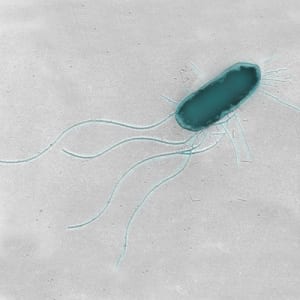Lots of classic applications of fluid dynamics are high Reynolds number flows, like aerodyamics or weather prediction. However, there are several very important applications of low Reynolds number flows. A particularly interesting one is determining why microorganisms swim differently to fish. Several bacteria have a long tail or flagellum that they use to propel themselves around. But how should they move this tail?
A fish can swim simply by flapping its tail backwards and forwards in a symmetric manner. But because bacteria are so tiny, the Reynolds number of the flow surrounding them is always low. Therefore if they flap their tail up, they will move forwards, but as they flap it down, the flow will reverse and they will move backwards by exactly the same amount. This is exactly the same thing that happens in our experiment, and it means bacteria have to work out another way to swim. They arrange their flagella in a corkscrew shape, and then rotate it, pushing the surrounding fluid backwards, and themselves forwards.
Or to put it another way, if you were a Borrower and you had to swim very slowly through some golden syrup, doggy paddle would be useless, but breaststroke would work a treat.
Anna Lambert and Oliver Southwick, both from UCL Mathematics, will be demonstrating the Taylor-Couette effect at the Spark Festival on 30-31 August.
This article is cross-posted from Chalkdust, a new mathematics magazine and blog written and edited by students from UCL’s mathematics department.
